comparing distribution of box plots Box plots visually show the distribution of numerical data and skewness by displaying the data quartiles (or percentiles) and averages. Box . CNC stands for Computer Numerical Control. This term can refer to a computer-based system that controls the motion of machine tools (machines) such as lathes and milling machines. In other words, it controls the machine tool’s ability to .
0 · side by boxplot interpretation
1 · matlab boxplot vs box chart
2 · how to solve box plots
3 · comparing box plots worksheet pdf
4 · comparing box plots worksheet
5 · comparing box plots problems
6 · comparing box plots and histograms
7 · comparing box and whisker plots
Silvery "gneiss" metal shingle is elegant on a pale celadon shingled house. A copper roof will outlast your heirs -- and so will the bill for installing it, if you can score the material. Copper is far more scarce and expensive than it was in its roof heyday, but its appeal is timeless.
Box plots are useful because they allow us to gain a quick understanding of the distribution of values in a dataset. They’re also useful for comparing two different datasets. When comparing two or more box plots, we can answer four different questions: 1. How do the .Often we create multiple box plots on one plot to compare the distribution of .Box plots are a useful way to compare two or more sets of data visually. In statistics, a box plot is used to provide a visual summary of data. The distribution of data is shown through the . Box plots visually show the distribution of numerical data and skewness by displaying the data quartiles (or percentiles) and averages. Box .
Box plots truly shine when comparing data distributions across different groups. Their compact design offers a neat summary of data, making it a breeze to compare distributional properties of the groups through the positioning of box .
Often we create multiple box plots on one plot to compare the distribution of several datasets at once. The following example shows how to compare the variability between several box plots in practice.
When comparing two or more box plots, we can answer four different questions: 1. How do the median values compare? We can compare the vertical line in each box to determine which dataset has a higher median . Box plots are useful for identifying outliers and for comparing distributions. We will explain box plots with the help of data from an in-class experiment. As part of the "Stroop Interference Case Study," students in .
This post describes box plots and shows their advantages. Box plots, also called box and whisker plots, are more useful than histograms for comparing distributions. When comparing two or more box plots, we can answer four different questions: 1. How do the median values compare? We can compare the vertical line in each box to determine which dataset has a higher median . Box plots are useful because they allow us to gain a quick understanding of the distribution of values in a dataset. They’re also useful for comparing two different datasets. When comparing two or more box plots, we can answer four different questions: 1. How do the median values compare?
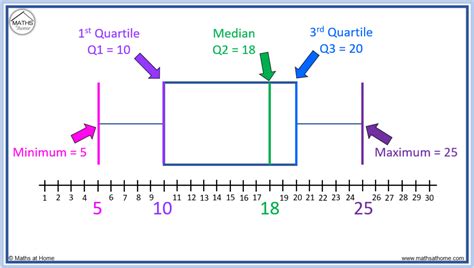
In this explainer, we will learn how to compare two data set distributions using box plots. Box plots, which are sometimes called box-and-whisker plots, can be a good way to visualize differences among groups that have been measured on the same variable.Box plots are a useful way to compare two or more sets of data visually. In statistics, a box plot is used to provide a visual summary of data. The distribution of data is shown through the positions of the median and the quartiles. From this, the spread and skew of the data can also be seen. Box plots visually show the distribution of numerical data and skewness by displaying the data quartiles (or percentiles) and averages. Box plots show the five-number summary of a set of data: including the minimum score, first (lower) quartile, median, third (upper) quartile, and maximum score.Box plots truly shine when comparing data distributions across different groups. Their compact design offers a neat summary of data, making it a breeze to compare distributional properties of the groups through the positioning of box and whisker markings.
Often we create multiple box plots on one plot to compare the distribution of several datasets at once. The following example shows how to compare the variability between several box plots in practice. When comparing two or more box plots, we can answer four different questions: 1. How do the median values compare? We can compare the vertical line in each box to determine which dataset has a higher median value. 2. How does the dispersion compare?
Box plots are useful for identifying outliers and for comparing distributions. We will explain box plots with the help of data from an in-class experiment. As part of the "Stroop Interference Case Study," students in introductory statistics were presented with a page containing \(30\) colored rectangles.
This post describes box plots and shows their advantages. Box plots, also called box and whisker plots, are more useful than histograms for comparing distributions.
When comparing two or more box plots, we can answer four different questions: 1. How do the median values compare? We can compare the vertical line in each box to determine which dataset has a higher median value. 2. How does the dispersion compare? Box plots are useful because they allow us to gain a quick understanding of the distribution of values in a dataset. They’re also useful for comparing two different datasets. When comparing two or more box plots, we can answer four different questions: 1. How do the median values compare?
international tool storage economy 19-in steel lockable tool box red
In this explainer, we will learn how to compare two data set distributions using box plots. Box plots, which are sometimes called box-and-whisker plots, can be a good way to visualize differences among groups that have been measured on the same variable.Box plots are a useful way to compare two or more sets of data visually. In statistics, a box plot is used to provide a visual summary of data. The distribution of data is shown through the positions of the median and the quartiles. From this, the spread and skew of the data can also be seen.
Box plots visually show the distribution of numerical data and skewness by displaying the data quartiles (or percentiles) and averages. Box plots show the five-number summary of a set of data: including the minimum score, first (lower) quartile, median, third (upper) quartile, and maximum score.Box plots truly shine when comparing data distributions across different groups. Their compact design offers a neat summary of data, making it a breeze to compare distributional properties of the groups through the positioning of box and whisker markings. Often we create multiple box plots on one plot to compare the distribution of several datasets at once. The following example shows how to compare the variability between several box plots in practice. When comparing two or more box plots, we can answer four different questions: 1. How do the median values compare? We can compare the vertical line in each box to determine which dataset has a higher median value. 2. How does the dispersion compare?
Box plots are useful for identifying outliers and for comparing distributions. We will explain box plots with the help of data from an in-class experiment. As part of the "Stroop Interference Case Study," students in introductory statistics were presented with a page containing \(30\) colored rectangles. This post describes box plots and shows their advantages. Box plots, also called box and whisker plots, are more useful than histograms for comparing distributions.
side by boxplot interpretation
ip junction box
intermatic junction box with transformer
into shiny metal boxes
interstate sheet metal
I heard that they don't offer prefab rocker panel parts for our cat, so I need to bend myself. What gauge sheet metal should I get? Once shaped, do I need to weld full, or 50% (for example)?
comparing distribution of box plots|side by boxplot interpretation